Producing multiple images of distant quasars or galaxies by foreground galaxies or galaxy clusters is one of the most distinct qualities of strong gravitational lensing. In our recent work, we have found that the cusp and the fold summations are not equal to 0, when a point source infinitely approaches a cusp or a fold from inner side of the caustic. Based on the magnification invariant theory, which states that the sum of signed magnifications of the total images of a given source is a constant, we calculate the cusp summations for the five lens models. We find that the cusp summations are always larger than 0 for source on the major cusps, while can be larger or smaller than 0 for source on the minor cusps. We also find that if these lenses tend to the circular lens, the major and minor cusp summations will have infinite values, and with positive and negative signs, respectively.   

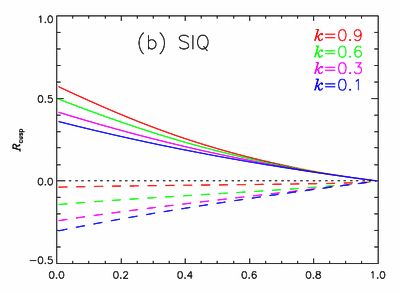
Figure 4. The solid and dashed curves show the cusp relations on the major and minor axes, respectively.  

    In addition, through the magnification invariants, we also derive the analytical signed cusp relations on the axes for three lens models. We find that both on the major and the minor axes the larger the lenses deviated from the circular lens, the larger the signed cusp relations. The major cusp relations are usually larger than the absolute minor cusp relations, but for some lens models with very large deviation from circular lens, the minor cusp relations can be larger than the major cusp relations.   

   The research work by Zhe Chu, Guoliang Li and Weipeng Lin (Sun Yat-Sen University) has been published in MNRAS.

  (http://mnras.oxfordjournals.org/content/449/2/2079).